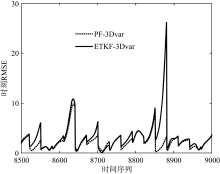
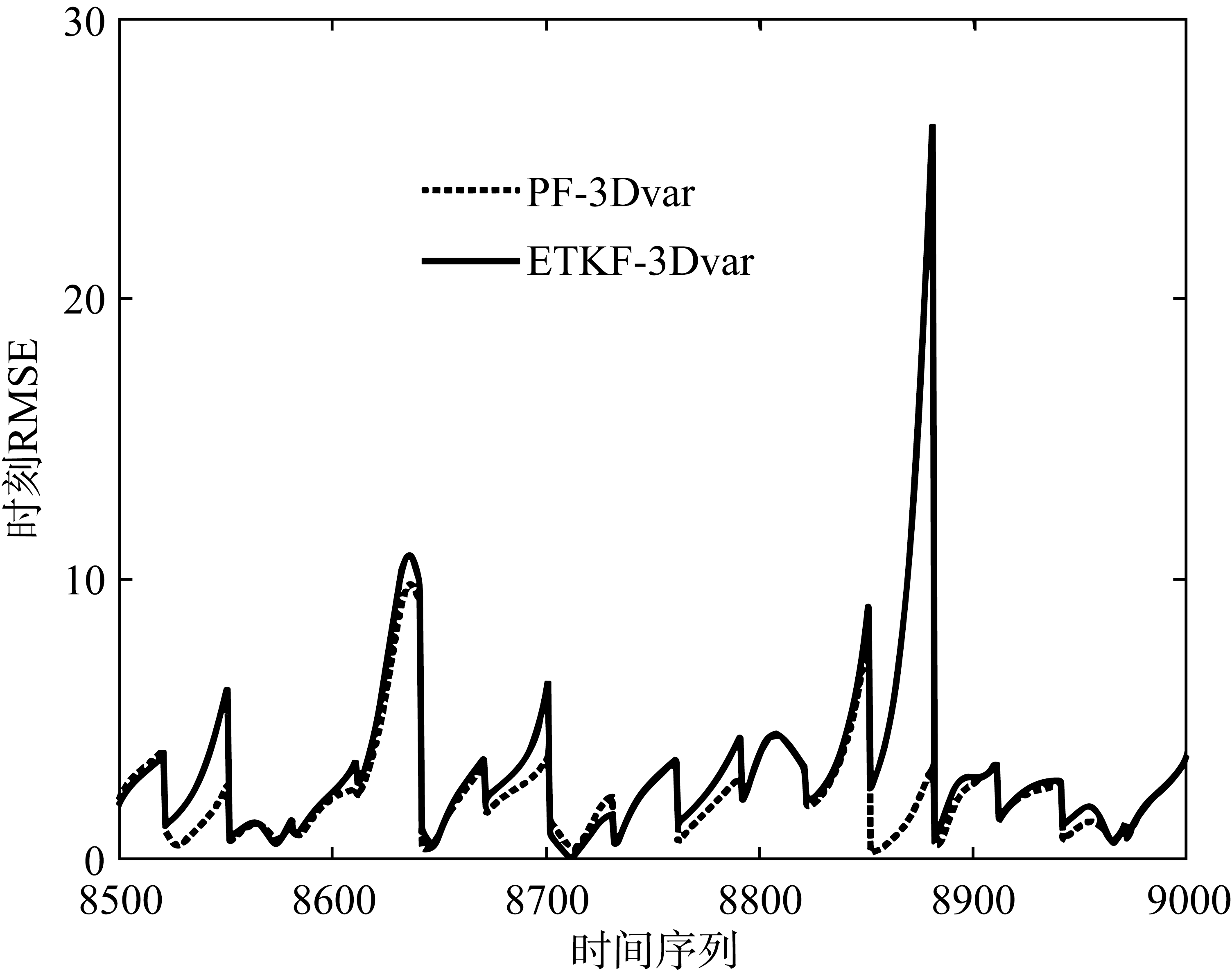
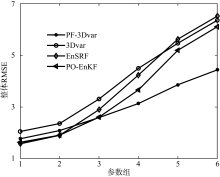
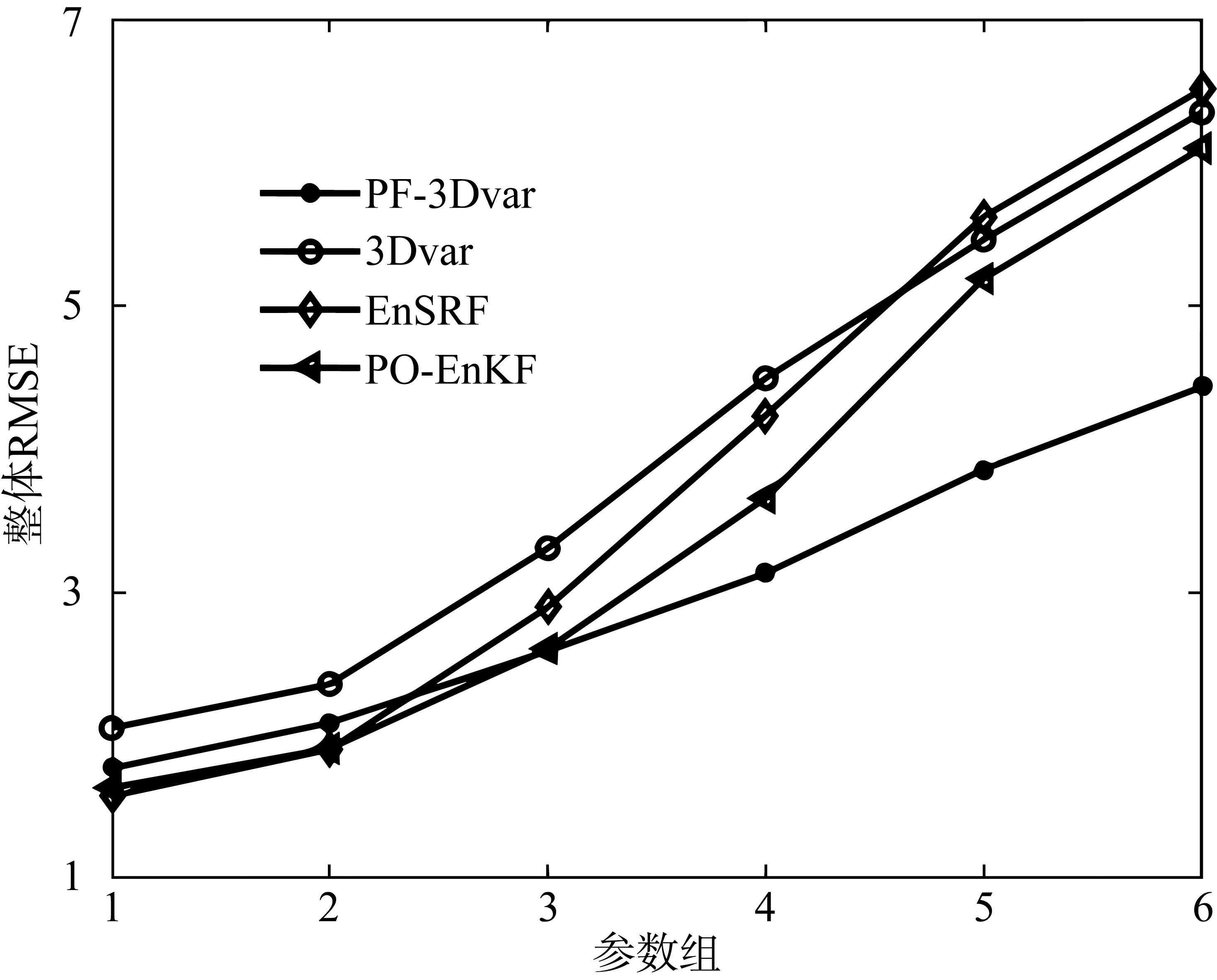
| [1] |
冯驰, 赵娜, 王萌, 2010. 一种改进残差重采样算法的研究[J]. 哈尔滨工程大学学报, 31(1): 120-124.
|
|
FENG CHI, ZHAO NA, WANG MENG, 2010. Improving the residual resampling algorithm[J]. Journal of Harbin Engineering University, 31(1): 120-124 (in Chinese with English abstract).
|
| [2] |
李新, 黄春林, 车涛, 2007. 中国陆面数据同化系统研究的进展与前瞻[J]. 自然科学进展, 17 (2): 163-173.
|
|
LI XIN, HUANG CHUNLIN, CHE TAO, et al, 2007. Progress and prospect of land surface data assimilation system in China[J]. Progress in Natural Science, (2): 163-173 (in Chinese with English abstract).
|
| [3] |
王法胜, 鲁明羽, 赵清杰, 等, 2014. 粒子滤波算法[J]. 计算机学报, 37(8): 1679-1694.
|
|
WANG FASHENG, LU MINGYU, ZHAO QINGJIE, et al, 2014. Particle filtering algorithm[J]. Chinese Journal of Computers, 37(8): 1679-1694 (in Chinese with English abstract).
|
| [4] |
吴新荣, 韩桂军, 李冬, 等, 2011. 集合滤波和三维变分混合数据同化方法研究[J]. 热带海洋学报, 30(6): 24-30.
doi: 10.11978/j.issn.1009-5470.2011.06.024
|
|
WU XINRONG, HAN GUIJUN, LI DONG, et al, 2011. A hybrid ensemble filter and 3D variational analysis scheme[J]. Journal of Tropical Oceanography, 30(6): 24-30 (in Chinese with English abstract).
|
| [5] |
ANDERSON J L, 2001. An ensemble adjustment Kalman filter for data assimilation[J]. Monthly Weather Review, 129(12): 2884-2903.
doi: 10.1175/1520-0493(2001)129<2884:AEAKFF>2.0.CO;2
|
| [6] |
ANDERSON J L, ANDERSON S L, 1999. A Monte Carlo implementation of the nonlinear filtering problem to produce ensemble assimilations and forecasts[J]. Monthly Weather Review, 127(12): 2741-2758.
doi: 10.1175/1520-0493(1999)127<2741:AMCIOT>2.0.CO;2
|
| [7] |
BISHOP C H, ETHERTON B J, MAJUMDAR S J, 2001. Adaptive sampling with the ensemble transform Kalman filter. part I: theoretical aspects[J]. Monthly Weather Review, 129(3): 420-436.
doi: 10.1175/1520-0493(2001)129<0420:ASWTET>2.0.CO;2
|
| [8] |
BURGERS G, VAN LEEUWEN P J, EVENSEN G, 1998. Analysis scheme in the ensemble Kalman filter[J]. Monthly Weather Review, 126(6): 1719-1724.
doi: 10.1175/1520-0493(1998)126<1719:ASITEK>2.0.CO;2
|
| [9] |
CARPENTER J, CLIFFORD P, FEARNHEAD P, 1999. Improved particle filter for nonlinear problems[J]. IEE Proceedings-Radar Sonar and Navigation, 146(1): 2-7.
doi: 10.1049/ip-rsn:19990255
|
| [10] |
EVENSEN G, 1994. Sequential data assimilation with a nonlinear quasi-geostrophic model using Monte Carlo methods to forecast error statistics[J]. Journal of Geophysical Research: Oceans, 99(C5): 10143-10162.
|
| [11] |
GOODLIFF M, AMEZCUA J, VAN LEEUWEN P J, 2015. Comparing hybrid data assimilation methods on the Lorenz 1963 model with increasing non-linearity[J]. Tellus A: Dynamic Meteorology and Oceanography, 67(1): 26928.
doi: 10.3402/tellusa.v67.26928
|
| [12] |
HAMILL T M, SNYDER C, 2000. A hybrid ensemble Kalman filter-3D variational analysis scheme[J]. Monthly Weather Review, 128(8): 2905-2919.
doi: 10.1175/1520-0493(2000)128<2905:AHEKFV>2.0.CO;2
|
| [13] |
HAMILL T M, WHITAKER J S, SNYDER C, 2001. Distance-dependent filtering of background error covariance estimates in an ensemble Kalman filter[J]. Monthly Weather Review, 129(11): 2776-2790.
doi: 10.1175/1520-0493(2001)129<2776:DDFOBE>2.0.CO;2
|
| [14] |
HUNT B R, KALNAY E, KOSTELICH E J, et al, 2004. Four-dimensional ensemble Kalman filtering[J]. Tellus A: Dynamic Meteorology and Oceanography, 56(4): 273-277.
doi: 10.3402/tellusa.v56i4.14424
|
| [15] |
KELLY D, MAJDA A J, TONG X T, 2015. Concrete ensemble Kalman filters with rigorous catastrophic filter divergence[J]. Proceedings of the National Academy of Sciences of the United States of America, 112(34): 10589-10594.
doi: 10.1073/pnas.1511063112
pmid: 26261335
|
| [16] |
KIM J, YOO J, DO K, 2020. Wave data assimilation to modify wind forcing using an ensemble Kalman filter[J]. Ocean Science Journal, 55(2): 231-247.
doi: 10.1007/s12601-020-0020-z
|
| [17] |
DIMET F-X L, TALAGRAND O, 2016. Variational algorithms for analysis and assimilation of meteorological observations: theoretical aspects[J]. Tellus A: Dynamic Meteorology and Oceanography, 38(2): 97-110.
doi: 10.3402/tellusa.v38i2.11706
|
| [18] |
LEITH C E, 1974. Theoretical skill of Monte Carlo forecasts[J]. Monthly Weather Review, 102(6): 409-418.
doi: 10.1175/1520-0493(1974)102<0409:TSOMCF>2.0.CO;2
|
| [19] |
LENG HONGZE, SONG JUNQIANG, 2013. Hybrid three-dimensional variation and particle filtering for nonlinear systems[J]. Chinese Physics B, 22(3): 030505.
doi: 10.1088/1674-1056/22/3/030505
|
| [20] |
LIU CHENGSI, XIAO QINGNONG, WANG BIN, 2008. An ensemble-based four-dimensional variational data assimilation scheme. part I: technical formulation and preliminary test[J]. Monthly Weather Review, 136(9): 3363-3373.
|
| [21] |
LORENC A C, 2003. The potential of the ensemble Kalman filter for NWP—a comparison with 4D‐Var[J]. Quarterly Journal of the Royal Meteorological Society, 129(595): 3183-3203.
|
| [22] |
MCLAUGHLIN D, 1995. Recent developments in hydrologic data assimilation[J]. Reviews of Geophysics, 33(S2): 977-984.
|
| [23] |
PRASAD V S, JOHNY J, SODHI J S, et al, 2016. Impact of EnVar hybrid assimilation using EnKF ensembles[C]. SPIE Asia-Pacific Remote Sensing, 98820J-98820J-10.
|
| [24] |
SOLBERGER M, SPÅNBERG E, 2020. Estimating a dynamic factor model in EViews Using the Kalman filter and smoother[J]. Computational Economics, 55(1): 875-900.
|
| [25] |
VEERAMALLA S K, TALARI V K H R, 2020. Multiple dipole source localization of EEG measurements using particle filter with partial stratified resampling[J]. Biomedical engineering letters, 10(2): 205-215.
|
| [26] |
WANG JIAN, GAO XIANG, CAO RUNAN, et al, 2021. A multilevel Monte Carlo method for performing time-variant reliability analysis[J]. IEEE Access, 9: 31773-31781
|
| [27] |
WANG XUGUANG, BARKER D M, SNYDER C, et al, 2007. A hybrid ETKF-3DVAR data assimilation scheme for the WRF Model. Part I: observing system simulation experiment[J]. Monthly Weather Review, 136(12): 5116-5131.
|
| [28] |
WHITAKER J S, HAMILL M, 2002. Ensemble data assimilation without perturbed observations[J]. Monthly Weather Review, 130(7): 1913-1924.
|
| [29] |
ZELTMANN S, MINOR A, OPHUS C, 2020. Denoising of sparse three- and four-dimensional hyperspectral electron microscopy data using a total variational method[J]. Microscopy and Microanalysis, 26(S2): 1724-1726.
|
| [30] |
ZHANG QINSHENG, TAGHVAEI A, CHEN YONGXIN, 2023. An optimal control approach to particle filtering[J]. Automatica, 151: 110894.
|
| [31] |
ZHOU WEI, LI JINGHUI, XU FANGHUA, et al, 2021. The impact of ocean data assimilation on seasonal predictions based on the National Climate Center climate system model[J]. Acta Oceanologica Sinica, 40(5): 58-70.
|
| [32] |
ZUPANSKI M, 2005. Maximum likelihood ensemble filter: theoretical aspects[J]. Monthly Weather Review, 133(6): 1710-1726.
|